Perpendicular Bisector
A perpendicular bisector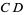 
of a line segment 
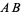is a line segment perpendicular to 
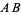and passing through the midpoint 
of 
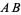(left figure). The perpendicular bisector of a line segment can be constructed using a compass by drawing circles centered at 
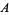and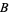 
with radius 
and connecting their two intersections. This line segment crosses 
at the midpoint 
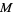of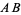 
(middle figure). If the midpoint 
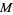is known, then the perpendicular bisector can be constructed by drawing a small auxiliary circle around 
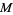, then drawing an arc from each endpoint that crosses the line 
at the farthest intersection of the circle with the line (i.e., arcs with radii 
and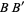 
respectively). Connecting the intersections of the arcs then gives the perpendicular bisector 
(right figure). Note that if the classical construction requirement that compasses be collapsible is dropped, then the auxiliary circle can be omitted and the rigid compass can be used to immediately draw the two arcs using any radius larger that half the length of 
.Angle Bisector
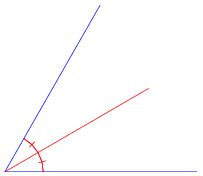
The (interior) bisector of an angle, also called the internal angle bisector (Kimberling 1998, pp. 11-12), is the line or line segment that divides the angle into two equal parts.
A. Constructing Perpendicular Bisector
B Angles Bisector
Google Form
No comments:
Post a Comment